A physical system and its dynamics can be described by mathematics. A few simple examples can be solved using pen and paper, but many real world problems are incredibly complex and require a computer to solve efficiently. Computers can perform thousands of mathematical operations per second, and therefore can solve complicated problems that would be impossible otherwise. Using a computer to solve maths that corresponds to a physical system is known as simulation.
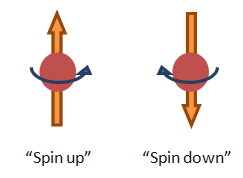
Quantum mechanics describes how the world works on atomic and sub-atomic scales, and since everything is made of atoms, it is the fundamental description of the universe. It predicts some strange and un-intuitive things, including the fact that something can be in two physical states at the same time, known as superposition. To understand superposition, imagine a quantum particle that can spin in two directions, one we call “spin up” and the other “spin down”, analogous to heads and tails in a coin. Superposition then means that the particle can be spin up and spin down at the same time. For two particles, there are four possible combinations of spins: up-up, down-down, up-down and down-up, all of which can be in a superposition. For three particles, there are eight combinations, for four there are sixteen combinations and the number of states increases exponentially with the number of particles. For 300 particles, there are more combinations than the number of atoms in the universe!
To simulate a quantum system, the phenomenon of superposition has to be taken into account. This is extremely bad for computers, as is evident from the spinning particle example. Even the world’s fastest supercomputers couldn’t perform non-trivial simulations involving more than 30 particles. And remember, these particles can only be in two physical states. Many real problems have more degrees of freedom, and physical quantities such as position and momentum can have infinitely many possibilities.
In 1982, famous quantum physicist Richard Feynman had the idea that instead of trying to simulate quantum systems with normal ‘classical’ computers, we could instead use a quantum system that can be controlled in the lab to simulate another quantum system that isn’t so easy to observe. This avoids the problem of the exponential increase in processing power with the number of particles, as quantum systems can be in a superposition and therefore automatically scale in the same way.
We use trapped ions as a controllable quantum system which can be used to simulate other quantum systems. The outer electron orbiting the atomic nucleus of the ion can be manipulated precisely using laser beams or microwaves, and can be made to spin in two different directions in the same way as in the quantum particle example. There are many exciting physical phenomena that can be investigated using this system. Two examples are being investigated in the group include 2d array of ion traps on a microfabricated chip to arrange the ions in a lattice structure. This arrangement mimics the structure of metals, in which spinning particles align to create permanent magnets, and there are many unsolved physical problems involving the dynamics of these spins that could be investigated using this experiment. Another simulation class makes use of a microfabricated chip trap where the ions are trapped in a ring configuration and can be moved around in a circle. This structure allows for the quantum simulation of Hawking radiation. Originally proposed by Stephen Hawking, the theory predicts that radiation should be emitted from the event horizon of a black hole. However, this radiation has never been observed.
There are a whole host of possible simulations that can be performed using trapped ions from all areas of physics, including effects of Einstein’s theory of special relativity, particle creation moments after the big bang and complex many-body phenomena such as quantum biology and quantum chemistry, all of which cannot be simulated on a classical computer. All of these examples provide unique research opportunities allowing trapped ion experiments to connect with problems from all areas of physics and science beyond.
